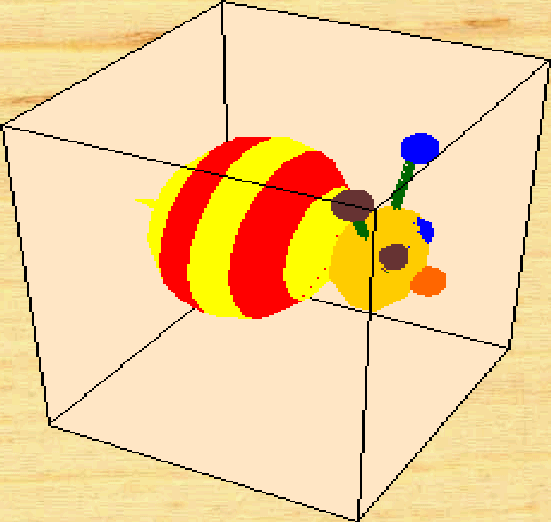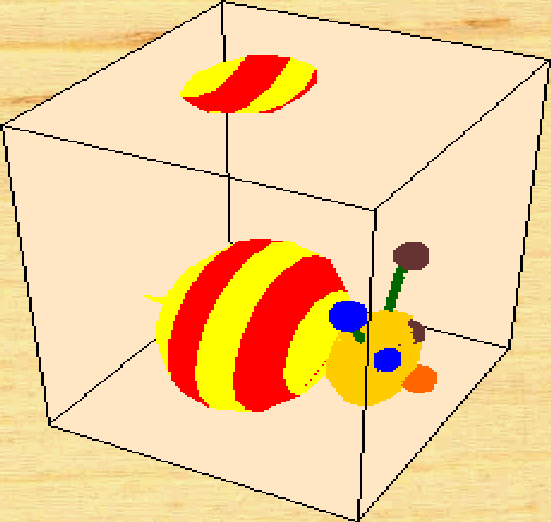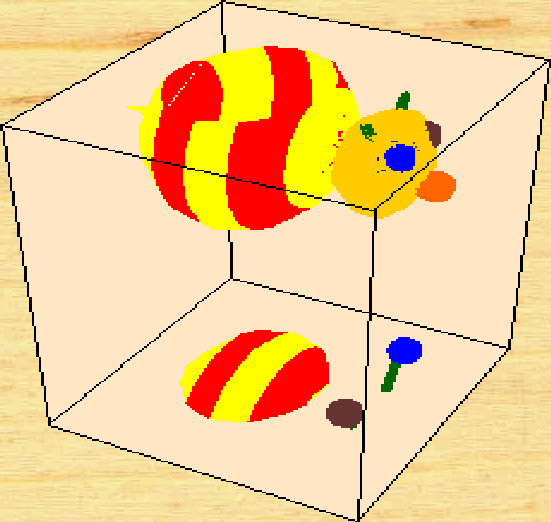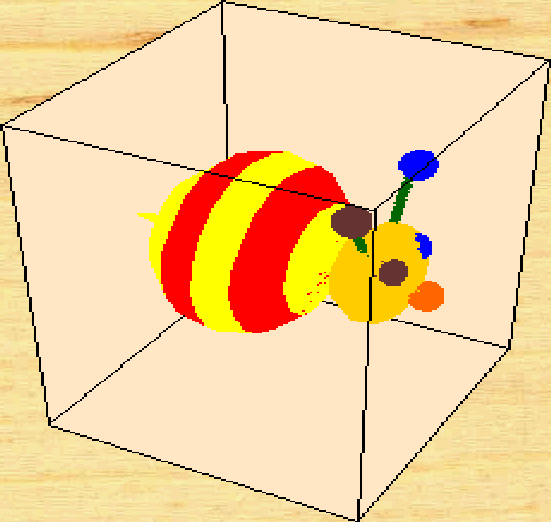
Traveling in a space obtained by identifying (in a special way) the opposite sides of the filled cube. This is a nonorientable space - look at the eyes of the bee.

Algebraic Topology is a study of topological objects through their algebraic invariants. Somewhat less formally, it is a study of spaces, including (in fact, mostly) those we can not perceive visually. The most important (for me) unsolved problem, classification of 3 manifolds (and the Poincare conjecture as a special case), is in the middle of the realm of Algebraic Topology. Very informally, 3 manifolds are spaces that locally feel like the space we live in. Poincare Conjecture states that the 3 sphere is deformable only to spaces that are essentially same as (=homeomorphic to) the 3 sphere itself. There is a 1 million price for the first solver.

Topics to be covered:

1. Homotopy Theory: Fundamental Groups, Homotopically Equivalent Spaces.

2. Group Theory: Presentations, Free Products, Free Products with Amalgamation.

3. The Seifert-Van Kampen Theorem: Computing Fundamental Groups of Spaces.

4. Covering Spaces: Covering Spaces, Covering Groups

5. Classification of coverings: Universal Coverings, Classification Theorems (time permitting)

6. Singular Homology (time permitting but unlikely).

Primary textbook: Introduction to Topological Manifolds, by John M. Lee. Secondary textbook: Introduction to Algebraic Topology, by Massey.

© S.Kalajdzievski