For every ![]() there exists a number N such that if
there exists a number N such that if ![]() then
then ![]() .
.
136.271
Midterm Exam 1
October 17, 2001
(50 minutes; justify your answers unless otherwise stated; no calculators)
1.
(a) State the definition of a convergent sequence. (What does it mean to say that
For every ![]() there exists a number N such that if
there exists a number N such that if ![]() then
then ![]() .
.
(b) Use only the definition in (a) to prove that
Let ![]() . We want to find N such that if
. We want to find N such that if ![]() then
then ![]() . The last inequality can be changed (without affecting its meaning) as follows:
. The last inequality can be changed (without affecting its meaning) as follows: ![]() means
means ![]() , means (since the term in the absolute value is positive)
, means (since the term in the absolute value is positive) ![]() , means
, means ![]() , means
, means ![]() . This is what we want to happen for certain numbers n (those larger than some N). Choose N to be any number larger than
. This is what we want to happen for certain numbers n (those larger than some N). Choose N to be any number larger than ![]() . Now we check if the conclusion in the definition of limit is satisfied. Suppose
. Now we check if the conclusion in the definition of limit is satisfied. Suppose ![]() . Then (since
. Then (since ![]() ) we have that
) we have that ![]() . But, as we saw above, the last inequality means the same as
. But, as we saw above, the last inequality means the same as ![]() and so the desired conclusion holds.
and so the desired conclusion holds.
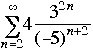 . Do not simplify your answer.
. Do not simplify your answer.
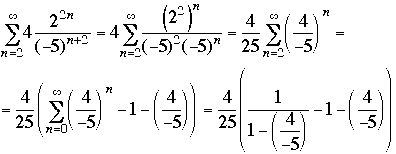
3. Show that the series
The following is a solution in which we only use the Simple Comparison Test; there are alternative simple solutions.
First note that all the terms are positive, so we can use any of the tests for convergence of positive series.
Since ![]() for
for ![]() , it follows that
, it follows that
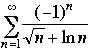 . Does it converge absolutely, does it converge conditionally, or does it diverge?
. Does it converge absolutely, does it converge conditionally, or does it diverge?
First we check for absolute convergence, that is, checking if
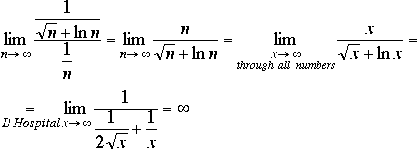 Since
Since So, the original series does not converge absolutely.
We check for conditional convergence — that is, we check if
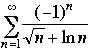 converges. This is obviously an alternating series. So, we may try the Alternating Series Test. For
converges. This is obviously an alternating series. So, we may try the Alternating Series Test. For
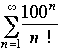
 . By the Ratio Test, the series converges.
. By the Ratio Test, the series converges.
used!).
The series is positive. We look at the function ![]() for
for ![]() . It is positive, continuous and decreasing (all are obvious). We have
. It is positive, continuous and decreasing (all are obvious). We have 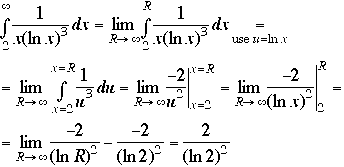
So we got a finite number. By the Integral Test, the original series converges.