2/3/4. 20 minutes
136.151 Test 3 Oct. 24 2000Student name: __ Student number: __
Value
[6] 1. Give an example of a function ![]() which is continuous everywhere but has no derivative at the point when
which is continuous everywhere but has no derivative at the point when ![]() . Provide a formula as well as the graph of your function.
. Provide a formula as well as the graph of your function.
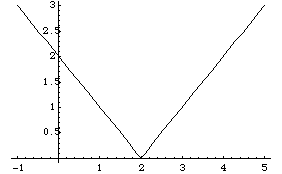
One example is ![]() . The graph of that function is given below:
. The graph of that function is given below:
[7] 2. (a) Find the second derivative ![]() of the function
of the function ![]() .
.
(b) Find ![]() if
if ![]() . Do not simplify.
. Do not simplify.
(a) ![]() ;
;
![]()
(b) ![]() ; so
; so ![]()
[7] 3. A particle is moving along the x-axis and the (signed) distance![]() from the origin is given by the formula
from the origin is given by the formula ![]() , where t is time measured in seconds.
, where t is time measured in seconds.
(a) What is the velocity of the particle at the moment when the time t is 2 seconds ? (Do not simplify your answer)
(b) Is the particle moving to the right (toward the larger positive distances) or to the left at that moment ? Justify your answer in one sentence.
(a) the velocity ![]() is
is ![]() . At the moment when t is 2 it is
. At the moment when t is 2 it is ![]() .
.
(b) Since the velocity at that moment is![]() and is positive, the particle moves to the right at that moment.
and is positive, the particle moves to the right at that moment.
[5] 4. Find ![]() if
if ![]() .
.
Using the Chain Rule, we get ![]()
Alternatively, one may use the Extended Power Rule.
total
[25]