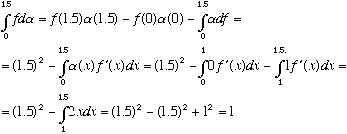[Recall that stands for the product ]

Hint: Consider the function .]

Denote . Since is continuous and since is a made from continuous functions by taking products and sums, it follows that is also continuous. The set is nothing but the inverse image of the set under . Since is continuous and since the set is closed, it follows that = is also closed.

2. Assume is continuous on [a,b] and has a finite second derivative in the open interval (a,b). Assume that the line segment joining the points and intersects the graph of in a third point P different from A and B. Prove that for some c in (a,b).

[This is question 5.19 in the text]

Suppose the point P is for some d in (a,b) (this is so since P lies on the graph of ). Since the points A, B and P lie on the same line, we have . By the Mean Value Theorem, there is a in (a,d) such that and there is a in (d,b) such that . So, . Since exists, the function is differentiable Role's can be applied to conclude that there is some c in such that .

3. The following 3 questions are mutually independent.

(a) Suppose is given by where is a decreasing function. Show that is of bounded total variation.

Since is monotonic, it is of a bounded variation (theorem). It follows that is of a bounded variation (as a product of two functions of bounded variation), and that is of bounded variation (as a sum of two such functions (theorem).

(b) Find one function such that is not of bounded variation over any subinterval of .

will do.

(c) Suppose is a rectifiable path. Show that the length of the curve in defined by satisfies . (Proof needed; a picture is not sufficient here.)

Take the following partition of [a,b]: . The length is the supremum of where runs through all partitions of [a,b]. Consequently, . On the other hand, .

4. Evaluate the Riemann-Stieltjes integral , or show it does not exist.

(a)      

The only dicontinuities of happens when x=1. Here, is continuous from right (discontinuous from left). At the same point, the function is continuous from left. By a theorem, it follows that .

(b)      

Note that here both and are dicontinuous form left. So, the theorem we have used in part (a) does not apply (but this does not tell us that the integral does not exist). Now, take a partition P of [a,b] containing the point x=1 (suppose in P). Then we have , for some in (since is zero elsewhere the other terms are anihilated). To simplify this a bit more, notice that , so that . The value of is either 0 or 1 depending on whether is chosen to be in or 1 respectively. Since any partition contains a rafinement containing the point x-1, it follows that does not exist.

(c) , .

Use integration by parts to get